Relationship between linear velocity and angular velocity

Table of Contents

Introduction:

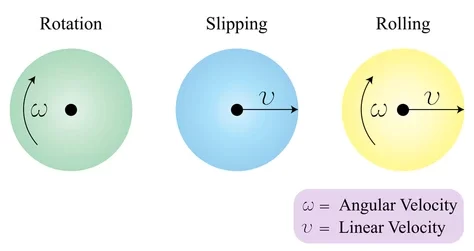
Movement is defined as a change in position over some period of time. In terms of physics and mechanics, this is called velocity. It is defined as a change in location over some time. Rotational motion is concerned with bodies moving around a fixed axis.

These bodies in the rotation session often give the behavior as the movements they showed during the transformation. For example, angular velocity and translational velocity are the same motion, as are torque-force and mass-moment of inertia.

Angular rotation:

Angular rotation is the rotation of a circle about a fixed axis. When working on the rotational problems, we try to draw parallels between transitional and rotational movements. The angle of rotation is defined by the angle through which the body moves. It is denoted by theta notation. When you do a rotation, the object that makes the circle move.

Arc length is the distance traveled by a rotating object. This distance and angular rotation are related to the radius of curvature. Let the length of the arc be denoted by “s” and the radius of curvature denoted by “r”.

θ = s/r

Angular velocity:

Suppose a body is rotating about a fixed axis. Therefore, the body changes angle over time. Expressing the angle by θ, the angular rate is defined as the rate of change of the angle of the body. Angular velocity is denoted by ω. If the body is rotating at a constant rate, the average angular velocity is used,

The instantaneous angular velocity is continuous when the rotational speed is not constant.

ω = dθ/DT

Angular Velocity and Linear Velocity:

Linear velocity is a measure of how fast an object is moving at a given time. For an object moving in a circle, the linear velocity is related to the angular velocity. An object that covers an angle also covers a certain distance on the arc of a circle. Suppose the line vector of a segment P rotating about a vertical axis is given by,

It is known

as s = rθ

Substituting the value of “s” in the permission given above,

The substitution of angular velocity values was saved in the previous section.

|v| = rω

As we know that a particle making circular motion can be fully determined by angular velocity, angular precision, etc. The movement of particles in circular motion is called circular motion. The displacement of the part along with the closure of the circle is determined according to the angle vector and the radius.

Angular displacement:

The angle subtended by the radius vector is known as angular displacement, expressed as θ, and has the SI unit radian.

If the particle completes one revolution of circular motion, then

Angular displacement = θ = 3600 = 2π rad

θ(in Rad) = Length of arc/radius = s/r

The instantaneous angular displacement is a vector quantity.

Its direction is given by the right-hand control and the magnitude in the vector is given as,

Angular velocity:

The rate of change of angular displacement with time is called angular velocity.

NO. Another unit is rad/s. Since the dimensions are [M0L0T-1θ1]

It is a multidimensional vector. Its direction is determined by the right-wing administration.

Let’s find the relationship between linear velocity and angular velocity:

Consider a piece with U.C.M of radius r clockwise from a horizontal circle of radius ‘r’.

Suppose a particle moves from point A to B. i.e. distance δs in short time δt. This distance is a straight line. The magnitude of the instantaneous velocity is given by,

The instantaneous velocity of the particle at any instant can be written as,

V = r (2π/T) = 2πr/T

Also 1/T = n (frequency)

V = 2 pr