Q: How is volumetric strain calculated?

Volumetric strain (ε) is calculated by dividing the change in volume (ΔV) by the original volume (V0) of the material and multiplying it by 100 to express it as a percentage: ε = (ΔV / V0) × 100.

Q: What are the units of volumetric strain?

Volumetric strain is a dimensionless quantity since it represents the relative change in volume. It is typically expressed as a percentage or a decimal.

Q: What are the causes of volumetric strain?

Volumetric strain can result from various factors, including thermal expansion or contraction, applied pressure, and mechanical deformation due to tension, compression, or shear.

Q: How does volumetric strain relate to other strain measures?

Volumetric strain is related to linear strain (εL) and shear strain (γ) through mathematical relationships. For example, volumetric strain can be calculated using the equation ε = εL + 2γ.

Q: What is the significance of volumetric strain in engineering?

Volumetric strain is crucial in engineering disciplines such as geotechnical engineering, structural analysis, and materials science, as it helps evaluate the behavior of materials under different loading conditions and assess their stability and performance.

Q: Can volumetric strain be positive or negative?

Yes, volumetric strain can be positive or negative depending on whether the material is expanding or contracting. Positive volumetric strain corresponds to an increase in volume, while negative volumetric strain represents a decrease in volume.

Q: How does temperature affect volumetric strain?

Temperature changes can cause volumetric strain due to thermal expansion or contraction. When a material is heated, it expands, resulting in positive volumetric strain, whereas cooling leads to negative volumetric strain.

Q: Is volumetric strain an elastic or plastic property?

Volumetric strain can occur in both elastic and plastic deformation. In elastic materials, volumetric strain is reversible, meaning the material returns to its original volume once the applied stress is removed. In plastic materials, the volumetric strain may be irreversible due to permanent deformation.

Q: How is volumetric strain measured experimentally?

Volumetric strain can be measured experimentally using various techniques, including strain gauges, extensometers, and displacement sensors, or by monitoring changes in the material's dimensions using optical or laser-based methods.

Volumetric strain – Definition, Significance & Applications

Table of Contents

Introduction:

Volumetric strain is a physical concept that describes the change in volume of an object or material due to an external force or pressure. It is an important topic in engineering and materials science, as it can have significant implications on the behavior and performance of structures and materials under various conditions.

Definition of Volumetric Strain

Volumetric strain is defined as “The change in volume of a material or object per unit volume, caused by an external force or pressure”. It is typically denoted by the symbol ε, and can be expressed as a decimal or a percentage. Volumetric strain is a measure of how much a material or object expands or contracts due to an external force and is directly related to its bulk modulus, which is a measure of its resistance to compression.

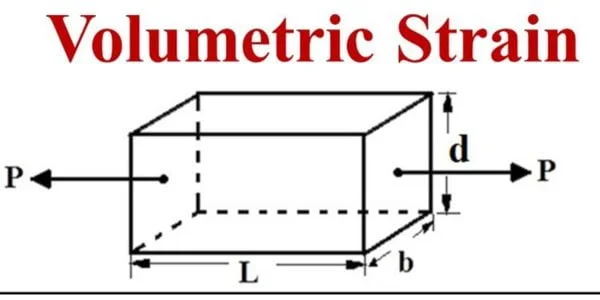
Calculation of Volumetric Strain

The formula for calculating volumetric strain is as follows:

ε = ΔV/V

Where ΔV is the change in volume of the material or object, and V is the original volume. The result is expressed as a decimal or a percentage.

For example, if a material with an original volume of 100 cubic meters experiences a change in volume of 5 cubic meters due to an external force, the volumetric strain would be:

ε = ΔV/V = 5/100 = 0.05 or 5%

The volumetric strain formula varies for different types of members.

The volumetric strain formula for three different types of members:

Rectangular bar,

Cylindrical rod,

Spherical body.

Rectangular Bar:

Derive the volumetric strain equation for a Rectangular bar:

A rectangular bar is a solid object that has a rectangular cross-section. When a rectangular bar is subjected to external forces or loads, it undergoes deformation in three directions. These three directions are along the x-axis, y-axis, and z-axis. The deformation along each of these axes is represented by the strains, εx, εy, and εz, respectively.

The volumetric strain, εv, is defined as the sum of the strains along all three axes. Therefore, the volumetric strain equation for a rectangular bar can be written as follows:

εv = εx + εy + εz

To derive the equation for volumetric strain, we need to first understand the definition of strain. Strain is defined as the change in length divided by the original length. Therefore, the strain along the x-axis, εx, can be defined as follows:

εx = ΔLx / Lx

where ΔLx is the change in length along the x-axis and Lx is the original length along the x-axis.

Similarly, the strains along the y-axis, εy, and z-axis, εz, can be defined as follows:

εy = ΔLy / Ly

εz = ΔLz / Lz

where ΔLy and ΔLz are the changes in length along the y-axis and z-axis, respectively, and Ly and Lz are the original lengths along the y-axis and z-axis, respectively.

Now, we need to find the change in volume of the rectangular bar, ΔV. The change in volume, ΔV, can be calculated by subtracting the original volume, V0, from the final volume, Vf.

ΔV = Vf – V0

The original volume, V0, of a rectangular bar can be calculated by multiplying the original length, width, and height of the bar.

V0 = Lx * Ly * Lz

Similarly, the final volume, Vf, can be calculated by multiplying the final length, width, and height of the bar.

Vf = (Lx + ΔLx) * (Ly + ΔLy) * (Lz + ΔLz)

Expanding the equation for Vf, we get:

Vf = LxLyLz + LxLyΔLz + LxΔLyLz + ΔLxLyLz + LxΔLyΔLz + ΔLxLyΔLz + ΔLxΔLyLz + ΔLxΔLyΔLz

Substituting the values of V0 and Vf in the equation for ΔV, we get:

ΔV = (LxLyLz + LxLyΔLz + LxΔLyLz + ΔLxLyLz + LxΔLyΔLz + ΔLxLyΔLz + ΔLxΔLyLz + ΔLxΔLyΔLz) – LxLyLz

Simplifying the above equation, we get:

ΔV = LxΔLyLz + ΔLxLyLz + LxLyΔLz + ΔLxΔLyLz + LxΔLy

Cylindrical Rod:

Derive the volumetric strain equation for a cylindrical rod.

Before we start, let’s understand the basic concept of volumetric strain. Volumetric strain is defined as the change in volume per unit volume of the material. It is represented by the symbol ε_v and is given by the equation:

ε_v = ΔV/V

Where ΔV is the change in volume, and V is the original volume of the material.

Now, let’s consider a cylindrical rod of length L, radius r, and volume V. Let us assume that the rod is subjected to an axial force F, causing a change in length ΔL. Due to this change in length, the volume of the rod will also change. Let us calculate the volumetric strain of the cylindrical rod due to the axial force F.

The original volume of the cylindrical rod can be calculated as:

V = πr^2L

After applying the axial force F, the rod will experience a change in length ΔL. The new length of the rod will be:

L’ = L + ΔL

The new volume of the rod can be calculated as:

V’ = πr^2L’

Substituting L’ in the above equation, we get:

V’ = πr^2(L + ΔL)

Expanding the equation, we get:

V’ = πr^2L + πr^2ΔL

The change in volume can be calculated as:

ΔV = V’ – V

Substituting the values of V’ and V, we get:

ΔV = πr^2L + πr^2ΔL – πr^2L

Simplifying the equation, we get:

ΔV = πr^2ΔL

Substituting the value of ΔV in the volumetric strain equation, we get:

ε_v = ΔV/V

Substituting the value of ΔV and V, we get:

ε_v = (πr^2ΔL)/(πr^2L)

Simplifying the equation, we get:

ε_v = ΔL/L

Therefore, the volumetric strain equation for a cylindrical rod is:

ε_v = ΔL/L

Spherical Body:

Derive the volumetric strain equation for a spherical body:

The volumetric strain equation:

The volumetric strain is defined as the change in volume divided by the original volume. Therefore, the equation for the volumetric strain can be written as:

εv = ΔV/V

Where εv is the volumetric strain, ΔV is the change in volume, and V is the original volume.

For a spherical body, the original volume can be expressed as:

V = (4/3)πr^3

Where r is the radius of the sphere.

To calculate the change in volume, let’s assume that the spherical body undergoes a uniform deformation. In other words, every point on the sphere experiences the same amount of deformation. Under this assumption, the change in volume can be expressed as:

ΔV = Vε

Where ε is the strain, which is the same in all directions due to the uniform deformation.

The strain can be expressed as the ratio of the change in length to the original length. For a spherical body, the original length is twice the radius, i.e., 2r. Therefore, the change in length can be expressed as:

ΔL = ε(2r)

Now, let’s consider a small element of the spherical body with a thickness of dr. The change in volume of this element can be expressed as:

dΔV = 4πr^2drΔL

Substituting the values of ΔL and V in terms of strain and radius, we get:

dΔV = 8πr^3εdr

Integrating this equation over the entire volume of the sphere, we get the total change in volume as:

ΔV = 8πε ∫r1r2 r^2 dr

Where r1 and r2 are the initial and final radii of the sphere, respectively.

Substituting the expression for the original volume, V, and the total change in volume, ΔV, in the equation for volumetric strain, we get:

εv = ΔV/V

εv = (8πε ∫r1r2 r^2 dr)/(4/3)πr^3

εv = 6ε(r2^3 – r1^3)/(r1^3)

Significance of Volumetric Strain

Volumetric strain is an important concept in materials science and engineering, as it can have significant implications on the behavior and performance of structures and materials under various conditions. For example, it can affect the strength, durability, and stability of buildings, bridges, and other structures, as well as the performance of materials used in manufacturing, such as metals, plastics, and ceramics.

The volumetric strain is an important factor to consider in geotechnical engineering, where it can affect the stability and behavior of soils and rocks. It can also be used to predict the behavior of fluids and gases in reservoir engineering, where it can affect the flow rates and pressure gradients in oil and gas reservoirs.

Applications of Volumetric Strain

The volumetric strain has many practical applications in various fields, including:

1. Geotechnical engineering: Volumetric strain is used to predict the behavior and stability of soils and rocks under different loading conditions. It can be used to determine the potential for landslides, soil settlement, and other geotechnical hazards.

2. Reservoir engineering: Volumetric strain is used to predict the behavior of fluids and gases in oil and gas reservoirs, which can help in the design and optimization of production operations.

3. Materials science and engineering: Volumetric strain is used to study the behavior and properties of materials under different loading conditions. It can be used to determine the strength, durability, and stability of materials used in manufacturing.

4. Structural engineering: Volumetric strain is used to design and optimize the performance of buildings, bridges, and other structures. It can be used to determine the potential for deformation, buckling, and failure under different loading conditions.

FAQ

Q: How is volumetric strain calculated?
Volumetric strain (ε) is calculated by dividing the change in volume (ΔV) by the original volume (V0) of the material and multiplying it by 100 to express it as a percentage: ε = (ΔV / V0) × 100.
Q: What are the units of volumetric strain?
Volumetric strain is a dimensionless quantity since it represents the relative change in volume. It is typically expressed as a percentage or a decimal.
Q: What are the causes of volumetric strain?
Volumetric strain can result from various factors, including thermal expansion or contraction, applied pressure, and mechanical deformation due to tension, compression, or shear.
Q: How does volumetric strain relate to other strain measures?
Volumetric strain is related to linear strain (εL) and shear strain (γ) through mathematical relationships. For example, volumetric strain can be calculated using the equation ε = εL + 2γ.
Q: What is the significance of volumetric strain in engineering?
Volumetric strain is crucial in engineering disciplines such as geotechnical engineering, structural analysis, and materials science, as it helps evaluate the behavior of materials under different loading conditions and assess their stability and performance.
Q: Can volumetric strain be positive or negative?
Yes, volumetric strain can be positive or negative depending on whether the material is expanding or contracting. Positive volumetric strain corresponds to an increase in volume, while negative volumetric strain represents a decrease in volume.
Q: How does temperature affect volumetric strain?
Temperature changes can cause volumetric strain due to thermal expansion or contraction. When a material is heated, it expands, resulting in positive volumetric strain, whereas cooling leads to negative volumetric strain.
Q: Is volumetric strain an elastic or plastic property?
Volumetric strain can occur in both elastic and plastic deformation. In elastic materials, volumetric strain is reversible, meaning the material returns to its original volume once the applied stress is removed. In plastic materials, the volumetric strain may be irreversible due to permanent deformation.
Q: How is volumetric strain measured experimentally?
Volumetric strain can be measured experimentally using various techniques, including strain gauges, extensometers, and displacement sensors, or by monitoring changes in the material’s dimensions using optical or laser-based methods.