Table of Contents
Shear Modulus: Understanding its Definition, Formula, and Importance
The shear modulus is a fundamental physical property that describes a material’s ability to resist deformation in response to applied shear stress. It is also known as the modulus of rigidity, and it is an essential parameter for characterizing the mechanical behavior of solid materials.
Definition of Shear Modulus
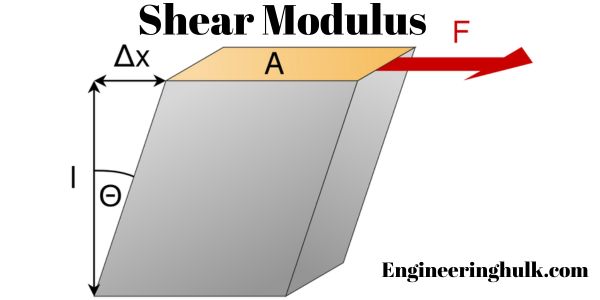
Shear modulus is defined as the ratio of shear stress to the corresponding shear strain in a material. In simple terms, it is a measure of a material’s resistance to deformation when subjected to an external force. It is denoted by the symbol G and expressed in units of pascals (Pa).
The formula for Shear Modulus
The formula for calculating shear modulus is given by:
G = τ/γ
G = (F/A)/(Δx/l)
G = Fl/AΔx
where G is the shear modulus, τ is the applied shear stress, and γ is the resulting shear strain.
where G is the shear modulus, F is the applied force, A is the cross-sectional area of the specimen, Δx is the displacement of the specimen, and l is the length of the specimen.
The unit of shear modulus
The unit of shear modulus is the pascal (Pa), which is equivalent to one newton per square meter (N/m²). The Pascal is a derived unit in the International System of Units (SI), and it is used to express the amount of stress required to produce a given amount of deformation.
Other units that can be used to express shear modulus include pounds per square inch (psi) and gigapascals (GPa). To convert from psi to Pa, you can use the conversion factor 1 psi = 6,894.76 Pa. To convert from GPa to Pa, you can use the conversion factor 1 GPa = 10^9 Pa.
Significance of Shear Modulus:
The shear modulus is a measure of the stiffness of a material when subjected to shear stress. It is an important material property that is used in the design of structural components, such as beams, shafts, and springs. The shear modulus is also used to calculate the shear strain in a material when subjected to shear stress.
The shear modulus is important in the study of elasticity, as it relates to the deformation of a material under shear stress. The shear modulus is related to Young’s modulus, which describes the material’s ability to withstand deformation under tensile or compressive stress.
Factors Affecting Shear Modulus:
The shear modulus of a material depends on various factors, including the material’s composition, structure, and temperature. Some materials, such as metals, have a higher shear modulus than others, such as plastics.
The crystalline structure of a material also affects its shear modulus. Materials with a regular, ordered structure, such as metals, have a higher shear modulus than materials with an amorphous, disordered structure, such as glass.
The temperature of a material also affects its shear modulus. As the temperature increases, the shear modulus of a material decreases. This is due to the increased mobility of the atoms in the material, which reduces its stiffness.
Importance and Application of Shear Modulus
Shear modulus is a critical material property that affects the behavior of materials in many applications. Some of the key areas where shear modulus plays a crucial role are:
1. Structural Engineering: In structural engineering, the shear modulus is used to calculate the shear deformation of beams, columns, and other structural elements. It is essential for designing structures that can withstand the stresses and strains of their intended use.
2. Material Science: In material science, the shear modulus is used to compare the mechanical properties of different materials. It is an important parameter for characterizing the strength and stiffness of materials, and it is often used in material testing and research.
3. Manufacturing: In manufacturing, the shear modulus is used to determine the optimal parameters for shaping and forming materials. By understanding the shear modulus of a material, manufacturers can choose the appropriate tools and techniques for shaping and processing the material.
4. Aerospace: In aerospace applications, the shear modulus is critical for designing components that can withstand the high stresses and strains of flight. It is used to determine the strength and stiffness of materials for use in aircraft structures, engines, and other critical components.
let’s consider an example to illustrate the use of this formula.
Shear modulus Examples:
Question 1. A rectangular block of steel is subjected to a shear stress of 200 MPa. The corresponding shear strain is 0.002. Calculate the shear modulus of the steel.
Solution:
Using the formula above, we can calculate the shear modulus of the steel as follows:
G = τ/γ
G = 200 MPa / 0.002
G = 100,000 MPa
Therefore, the shear modulus of the steel block is 100,000 MPa.
In this example, we have shown how to calculate shear modulus using the relevant formula. It is important to note that the value of shear modulus varies for different materials and can be affected by factors such as temperature, pressure, and composition. Shear modulus is an important property in the study of material science and is used to characterize the behavior of materials under shear stress.
Question 2. Consider a rectangular block made of steel with dimensions of 10 cm x 20 cm x 30 cm. The block is subjected to a shear stress of 200 MPa. Calculate the shear modulus of the steel block.
Solution:
The shear modulus of a material is defined as the ratio of shear stress to shear strain. Therefore, we need to calculate the shear strain in the block to determine the shear modulus. Let us assume that the block is sheared in the x-y plane, as shown in the figure below:
In this case, the shear strain (γ) can be calculated using the formula:
γ = tan θ
where θ is the angle of deformation. In the given figure, θ can be calculated as:
θ = L/h = 20/10 = 2
Therefore, the shear strain can be calculated as:
γ = tan θ = tan 2 = 1.10
Now, we can calculate the shear modulus (G) using the formula:
G = τ/γ
where τ is the shear stress. In this case, τ = 200 MPa. Substituting the values, we get:
G = 200/1.10 = 181.82 MPa
Therefore, the shear modulus of the steel block is 181.82 MPa.
Also, read the spring constant