Table of Contents
Introduction:
Young’s modulus of elasticity, also known as the modulus of elasticity, is a mechanical property that describes a material’s ability to resist deformation under an applied force. This property is an essential parameter for engineers and scientists involved in designing and analyzing structures and materials.
What is Young’s Modulus of Elasticity?
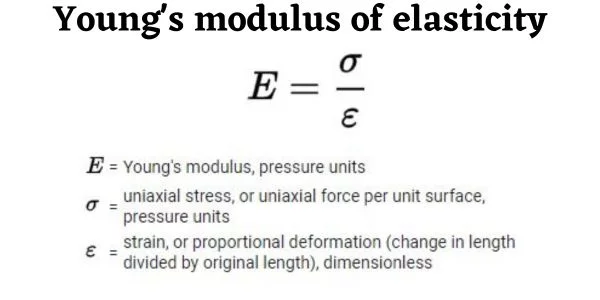
Young’s modulus of elasticity is defined as “The ratio of the stress applied to a material to the resulting strain produced”. It is denoted by the symbol “E” and is expressed in units of pressure, such as pascals (Pa) or pounds per square inch (psi). The equation for Young’s modulus is as follows:
The formula for calculating the modulus of elasticity is:
E = σ / ε
Where:
E = Modulus of elasticity (in Pa or N/m²)
σ = Applied stress (in Pa or N/m²)
ε = Strain produced (unitless)
The stress is defined as the force per unit area applied to a material, and the strain is the amount of deformation that occurs in response to the stress. Young’s modulus is a measure of a material’s resistance to elastic deformation, meaning the ability to return to its original shape after being subjected to stress.
Strain is Change in Length/Original Length
ε = Δl/l
As we know,
Stress = Force/Area
σ = F/A
E = σ/ε
E = (F/A)/(Δl/l)
E = (F × l)/(Δl × A)
Calculation
To calculate the modulus of elasticity, we must first measure the stress and strain produced in the material under a known load. This can be done using a variety of testing methods, including tension, compression, and bending tests.
In a tension test, a material sample is pulled apart until it breaks. The amount of force required to break the sample is recorded, along with the change in length of the sample. The stress is calculated by dividing the force by the cross-sectional area of the sample, while the strain is calculated by dividing the change in length by the original length of the sample. These values can then be used to calculate the modulus of elasticity using the formula above.
In a compression test, a material sample is compressed until it deforms. The force required to achieve a certain amount of deformation is recorded, along with the change in the length of the sample. The stress and strain are calculated in the same way as in a tension test, and the modulus of elasticity can be calculated using the same formula.
In a bending test, a material sample is placed on two supports, and a load is applied at the center of the sample. The amount of deflection of the sample is measured, along with the length and cross-sectional area of the sample. The stress and strain can be calculated using equations specific to bending, and the modulus of elasticity can be calculated using the same formula as before.
Importance of Young’s Modulus of Elasticity
Young’s modulus of elasticity is an important mechanical property for several reasons. It can be used to predict the behavior of a material under a variety of loading conditions, such as tension, compression, and bending.
Engineers use Young’s modulus to design and analyze structures and components, such as bridges, buildings, and aircraft. Understanding a material’s Young’s modulus is also important for selecting appropriate materials for specific applications.
Factors Affecting Elasticity
Factor #1: Material Composition
The composition of a material has a significant impact on Young’s modulus. Materials that are composed of tightly packed, ordered structures, such as metals, have high Young’s moduli. On the other hand, materials with disordered structures, such as polymers, have lower Young’s moduli.
The stiffness of a material is related to the strength of the chemical bonds that hold its atoms or molecules together. Therefore, materials with stronger chemical bonds have higher Young’s moduli.
Factor #2: Temperature
Temperature is another factor that affects Young’s modulus. As the temperature of a material increases, its atoms or molecules vibrate more vigorously, which can cause the material to become less stiff. This effect is particularly pronounced in polymers, which are known to exhibit a significant reduction in stiffness as the temperature increases. Metals, on the other hand, typically become stiffer at higher temperatures.
Factor #3: Strain Rate
The rate at which a material is strained also affects its Young’s modulus. When a material is subjected to a force, it deforms or stretches. The rate at which this deformation occurs can impact the material’s stiffness. In general, materials exhibit a higher Young’s modulus at lower strain rates. This is because slower deformation allows more time for the material’s internal structure to adjust and respond to the applied force.
Factor #4: Crystallinity
The degree of crystallinity in a material is also an important factor in its Young’s modulus. Crystalline materials, which have highly ordered structures, typically have higher Young’s moduli than amorphous materials, which lack a well-defined structure. This is because the ordered structure of crystalline materials provides a more direct pathway for the transmission of forces through the material.
Factor #5: Moisture Content
The presence of moisture can also impact a material’s Young’s modulus. When a material absorbs moisture, it can cause the material to swell, which can reduce its stiffness. This effect is particularly pronounced in polymers, which are known to be highly sensitive to moisture.
In some cases, the presence of moisture can cause a material to exhibit a significant reduction in stiffness, which can impact its performance in certain applications.
Applications of Young’s Modulus of Elasticity
Young’s modulus of elasticity has several applications in engineering and science. Some of these applications include:
1. Design and analysis of structures:
Engineers use Young’s modulus to design and analyze structures, such as bridges, buildings, and aircraft. By knowing Young’s modulus of a material, they can predict how it will behave under different loading conditions, such as tension, compression, and bending.
2. Material selection:
Young’s modulus is an essential parameter for selecting appropriate materials for specific applications. For example, materials with a high Young’s modulus are suitable for applications that require high stiffness and strength, such as aerospace and automotive applications. Materials with a low Young’s modulus are suitable for applications that require flexibility and elasticity, such as soft robotics and medical devices.
3. Material characterization:
Young’s modulus is used to characterize the mechanical properties of materials. By measuring Young’s modulus of a material, scientists and engineers can determine its strength, stiffness, and elasticity.
4. Testing materials:
Young’s modulus is used to test the mechanical properties of materials. By subjecting a material to known stress and measuring the resulting strain, researchers can determine the material’s Young’s modulus and other mechanical properties.
Value of Young’s Modulus for Different Materials:
The value of Young’s modulus varies depending on the material. Here are some common materials and their respective values of Young’s modulus:
Steel: Young’s modulus of steel ranges from 190 to 210 GPa, depending on the grade and composition of the steel. Steel is known for its high strength and stiffness, making it a popular material for construction and engineering applications.
Aluminum: Young’s modulus of aluminum is approximately 70 GPa. Aluminum is a lightweight material with good corrosion resistance and thermal conductivity, making it popular for use in the aerospace industry.
Copper: Young’s modulus of copper is approximately 120 GPa. Copper is known for its high electrical and thermal conductivity, making it a popular material for electrical wiring and heat exchangers.
Glass: Young’s modulus of glass ranges from 50 to 90 GPa, depending on the type of glass. Glass is known for its transparency and high strength, making it popular for use in windows and optical components.
Rubber: Young’s modulus of rubber is typically less than 1 GPa. Rubber is known for its high elasticity and flexibility, making it popular for use in gaskets and seals.
Solved Examples
Example 1:
A steel bar with a cross-sectional area of 10 mm^2 is subjected to a tensile load of 500 N. The length of the bar is 2 meters, and after the load is applied, the length of the bar increases to 2.002 meters. Calculate Young’s modulus of the steel bar.
Solution:
Young’s modulus, E = stress/strain
stress = Force/Area = 500 N / 10 mm^2 = 50 MPa
strain = (final length – initial length) / initial length = (2.002 m – 2 m) / 2 m = 0.001
Young’s modulus = stress/strain = 50 MPa / 0.001 = 50,000 MPa
Therefore, Young’s modulus of the steel bar is 50,000 MPa.
Example 2:
A copper wire with a diameter of 1 mm and a length of 10 meters is stretched by a force of 100 N. After stretching, the wire becomes 10.01 meters long. Calculate Young’s modulus of copper.
Solution:
Young’s modulus, E = stress/strain
stress = Force/Area = 100 N / ((pi/4)*(1 mm)^2) = 127,234.5 kPa
strain = (final length – initial length) / initial length = (10.01 m – 10 m) / 10 m = 0.001
Young’s modulus = stress/strain = 127,234.5 kPa / 0.001 = 127,234,500 Pa
Therefore, Young’s modulus of copper is 127,234,500 Pa.
Example 3:
A steel wire with a diameter of 2 mm and a length of 5 meters is suspended vertically from a fixed point. A weight of 500 N is hung from the lower end of the wire, causing it to stretch by 5 mm. Calculate Young’s modulus of steel.
Solution:
Young’s modulus, E = stress/strain
stress = Force/Area = 500 N / ((pi/4)*(2 mm)^2) = 198,943.5 kPa
strain = (final length – initial length) / initial length = 5 mm / 5 m = 0.001
Young’s modulus = stress/strain = 198,943.5 kPa / 0.001 = 198,943,500 Pa
Therefore, Young’s modulus of steel is 198,943,500 Pa.
Also, read the milling cutter